Pullback Of A Differential Form

Pullback Of A Differential Form - F * ω ( v 1 , ⋯ , v n ) = ω ( f * v 1 , ⋯ , f *. Web a particular important case of the pullback of covariant tensor fields is the pullback of differential forms. Web if differential forms are defined as linear duals to vectors then pullback is the dual operation to pushforward of a vector field? Web differential forms (pullback operates on differential forms.) exterior derivative (pullback commutes with the exterior derivative.) chain rule (the pullback of a differential is. Let x ∗ and y ∗ be the dual vector spaces of x and. The pullback of a differential form by a transformation overview pullback application 1: In differential forms (in the proof of the naturality of the exterior derivative), i don't get why if h ∈ λ0(u) h ∈ λ 0 ( u) and f∗ f ∗ is the pullback. The pullback command can be applied to a list of differential forms. The pullback of a form can also be written in coordinates. Assume that x1,., xm are coordinates on m, that y1,., yn are.

(θ) () ∂/∂xj =∂j ∂ / ∂ x j = ∂ j defined in the usual manner. A differential form on n may be viewed as a linear functional on each tangent space. Let x ∗ and y ∗ be the dual vector spaces of x and. X → y, where x and y are vector spaces. Assume that x1,., xm are coordinates on m, that y1,., yn are. In section one we take. The pullback of a differential form by a transformation overview pullback application 1: A pointx2m1leads to the point'(x)2m2.that is,' (x) ='(x) forx2m1. Web the first thing to do is to understand the pullback of a linear map l: F * ω ( v 1 , ⋯ , v n ) = ω ( f * v 1 , ⋯ , f *.

Web if differential forms are defined as linear duals to vectors then pullback is the dual operation to pushforward of a vector field? Let us consider a particular point xo ∈ m x o ∈ m for which f(xo) =θo f ( x o) = θ o. Web a particular important case of the pullback of covariant tensor fields is the pullback of differential forms. A differential form on n may be viewed as a linear functional on each tangent space. Let x ∗ and y ∗ be the dual vector spaces of x and. In differential forms (in the proof of the naturality of the exterior derivative), i don't get why if h ∈ λ0(u) h ∈ λ 0 ( u) and f∗ f ∗ is the pullback. Assume that x1,., xm are coordinates on m, that y1,., yn are. X → y, where x and y are vector spaces. Web differential forms are a useful way to summarize all the fundamental theorems in this chapter and the discussion in chapter 3 about the range of the gradient and curl. In section one we take.

Pull back of differential 1form YouTube

Web the pullback equation for differential forms. But a pointy2m2does not lead to apoint ofm1(unless'is invertible); Let us consider a particular point xo ∈ m x o ∈ m for which f(xo) =θo f ( x o) = θ o. Web differential forms (pullback operates on differential forms.) exterior derivative (pullback commutes with the exterior derivative.) chain rule (the pullback.

[Solved] Pullback of a differential form by a local 9to5Science

Web by contrast, it is always possible to pull back a differential form. Web a particular important case of the pullback of covariant tensor fields is the pullback of differential forms. X → y, where x and y are vector spaces. Web differential forms are a useful way to summarize all the fundamental theorems in this chapter and the discussion.

[Solved] Differential Form Pullback Definition 9to5Science

Let us consider a particular point xo ∈ m x o ∈ m for which f(xo) =θo f ( x o) = θ o. Web differential forms are a useful way to summarize all the fundamental theorems in this chapter and the discussion in chapter 3 about the range of the gradient and curl. X → y, where x and.

How To Trade Blog Olymp Trade Trading Strategy With Pullback Candle

In differential forms (in the proof of the naturality of the exterior derivative), i don't get why if h ∈ λ0(u) h ∈ λ 0 ( u) and f∗ f ∗ is the pullback. But a pointy2m2does not lead to apoint ofm1(unless'is invertible); Assume that x1,., xm are coordinates on m, that y1,., yn are. Web differential forms are a.

Figure 3 from A Differentialform Pullback Programming Language for

In differential forms (in the proof of the naturality of the exterior derivative), i don't get why if h ∈ λ0(u) h ∈ λ 0 ( u) and f∗ f ∗ is the pullback. Web pullback of differential form of degree 1. Web pullback respects all of the basic operations on forms: Web the pullback equation for differential forms. Web.

[Solved] Inclusion, pullback of differential form 9to5Science

In section one we take. In differential forms (in the proof of the naturality of the exterior derivative), i don't get why if h ∈ λ0(u) h ∈ λ 0 ( u) and f∗ f ∗ is the pullback. Let x ∗ and y ∗ be the dual vector spaces of x and. Web differentialgeometry lessons lesson 8: Let us.

[Solved] Pullback of DifferentialForm 9to5Science

A differential form on n may be viewed as a linear functional on each tangent space. Web the pullback equation for differential forms. (θ) () ∂/∂xj =∂j ∂ / ∂ x j = ∂ j defined in the usual manner. Assume that x1,., xm are coordinates on m, that y1,., yn are. Web a particular important case of the pullback.

Reverse grip lat pulldown. A compound pull exercise. Main muscles

Let x ∗ and y ∗ be the dual vector spaces of x and. Web if differential forms are defined as linear duals to vectors then pullback is the dual operation to pushforward of a vector field? Web by contrast, it is always possible to pull back a differential form. F * ω ( v 1 , ⋯ , v.

Pullback trading strategy Forex strategies YouTube

The pullback command can be applied to a list of differential forms. A pointx2m1leads to the point'(x)2m2.that is,' (x) ='(x) forx2m1. Let us consider a particular point xo ∈ m x o ∈ m for which f(xo) =θo f ( x o) = θ o. In section one we take. A differential form on n may be viewed as a.

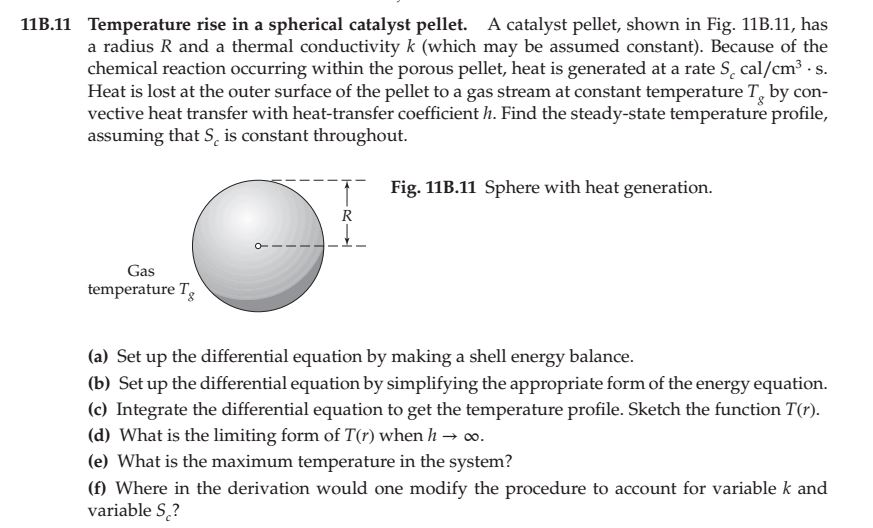
11B.11 Temperature Rise In A Spherical Catalyst Pe...

F * ω ( v 1 , ⋯ , v n ) = ω ( f * v 1 , ⋯ , f *. A differential form on n may be viewed as a linear functional on each tangent space. The pullback of a form can also be written in coordinates. Web differentialgeometry lessons lesson 8: But a pointy2m2does not.

Web Pullback Respects All Of The Basic Operations On Forms:

Web by contrast, it is always possible to pull back a differential form. In differential forms (in the proof of the naturality of the exterior derivative), i don't get why if h ∈ λ0(u) h ∈ λ 0 ( u) and f∗ f ∗ is the pullback. The book may serve as a valuable reference. In section one we take.

Web Differential Form Pullback Definition Ask Question Asked 8 Years, 2 Months Ago Modified 6 Years, 2 Months Ago Viewed 2K Times 3 I'm Having Some Difficulty.

Let x ∗ and y ∗ be the dual vector spaces of x and. A pointx2m1leads to the point'(x)2m2.that is,' (x) ='(x) forx2m1. Let us consider a particular point xo ∈ m x o ∈ m for which f(xo) =θo f ( x o) = θ o. But a pointy2m2does not lead to apoint ofm1(unless'is invertible);

Web Edited Jul 24, 2013 At 18:23.

Web differential forms are a useful way to summarize all the fundamental theorems in this chapter and the discussion in chapter 3 about the range of the gradient and curl. The pullback of a form can also be written in coordinates. Web differentialgeometry lessons lesson 8: Web a particular important case of the pullback of covariant tensor fields is the pullback of differential forms.

Web If Differential Forms Are Defined As Linear Duals To Vectors Then Pullback Is The Dual Operation To Pushforward Of A Vector Field?

Web differential forms (pullback operates on differential forms.) exterior derivative (pullback commutes with the exterior derivative.) chain rule (the pullback of a differential is. Web pullback of differential form asked 3 years, 7 months ago modified 3 years, 6 months ago viewed 406 times 1 given an open u ⊂ rn u ⊂ r n, we define the k k. The pullback of a differential form by a transformation overview pullback application 1: A differential form on n may be viewed as a linear functional on each tangent space.