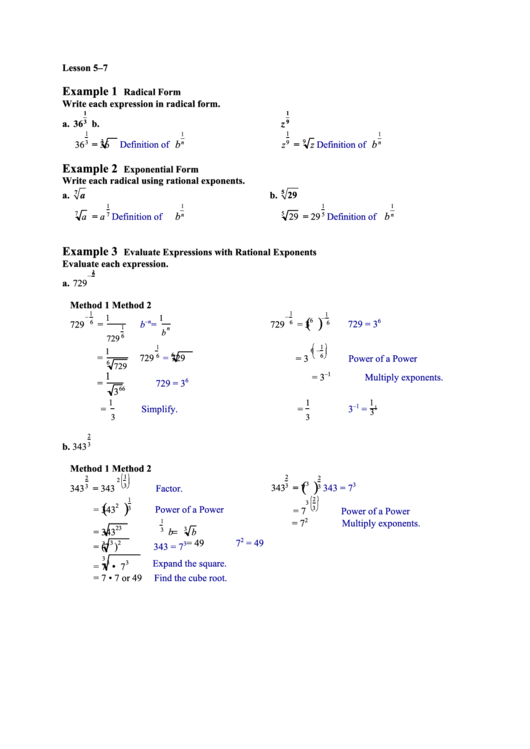
Write Each Expression In Radical Form
Write Each Expression In Radical Form - Web a worked example of simplifying an expression that is a sum of several radicals. 👉 learn how to convert a rational power to a radical. = 3 ⋅ x = 3x. Web simplifying radical expressions an algebraic expression that contains radicals is called a radical expression14. When the exponent of an expression is a fraction, we can evaluate/simplify the expression by converting the rational power. 3√27x3 = 3√33 ⋅ x3 applytheproductruleforradicals. 1) 7 1 22) 4 4 3 3) 2 5 34) 7 4 3 5) 6 3 26) 2 1 6 write each expression in exponential form. Let’s begin by building some fundamental skills. Solution use the fact that n√an = a when n is odd. There are no fractions in the radicand.
= 3√33 ⋅ 3√x3 simplify. Web simplifying radical expressions an algebraic expression that contains radicals is called a radical expression14. Sal rewrites (r^ (2/3)s^3)^2*√ (20r^4s^5), once as an exponential expression and once as a radical expression. 7) (10)38) 6 2 9) ( 4 2)510) (45)5 11) 3 2 12) 6 10 write each expression in radical form. Then, √a√b = √ab, and, provide b ≠ 0, √a √b = √a b in this section, we will simplify a number of more extensive expressions containing square roots, particularly those that are fundamental to your work in future mathematics courses. Click the blue arrow to submit. Solution use the fact that n√an = a when n is odd. = 3 ⋅ x = 3x. 👉 learn how to convert a rational power to a radical. Choose convert to radical form from the topic selector and click to see the result in our algebra calculator !
In this example, we simplify √(2x²)+4√8+3√(2x²)+√8. Web write each expression in radical form. We use the product and quotient rules to simplify them. Sal rewrites (r^ (2/3)s^3)^2*√ (20r^4s^5), once as an exponential expression and once as a radical expression. Click the blue arrow to submit. 👉 learn how to convert a rational power to a radical. Let’s begin by building some fundamental skills. Created by sal khan and monterey institute for technology and education. 1) 7 1 22) 4 4 3 3) 2 5 34) 7 4 3 5) 6 3 26) 2 1 6 write each expression in exponential form. Web this is because there will never be more than one possible answer for a radical with an odd index.
Write each expression in simplest radical form. I…
Click the blue arrow to submit. Enter the expression you want to convert into the radical form. 1) 7 1 22) 4 4 3 3) 2 5 34) 7 4 3 5) 6 3 26) 2 1 6 write each expression in exponential form. The radical index is as small as possible. Web a worked example of simplifying an expression.
Write each expression in simplest radical form. I…
Created by sal khan and monterey institute for technology and education. We use the product and quotient rules to simplify them. \[{a^{\frac{m}{n}}} = {\left( {{a^{\frac{1}{n}}}} \right)^m} = {\left( {\sqrt[n]{a}} \right)^m}\hspace{0.25in}\hspace{0.25in}{\mbox{or}}\hspace{0.25in}\hspace{0.25in. = 3√33 ⋅ 3√x3 simplify. 3√27x3 = 3√33 ⋅ x3 applytheproductruleforradicals.
Write each expression in simplest radical form. I…
Created by sal khan and monterey institute for technology and education. 👉 learn how to convert a rational power to a radical. = 3 ⋅ x = 3x. Click the blue arrow to submit. Let’s begin by building some fundamental skills.
[ANSWERED] Write each expression in radical form. Use "sqrt(x)" fo
3√27x3 = 3√33 ⋅ x3 applytheproductruleforradicals. The radical index is as small as possible. Solution use the fact that n√an = a when n is odd. 7) (10)38) 6 2 9) ( 4 2)510) (45)5 11) 3 2 12) 6 10 write each expression in radical form. Web this is because there will never be more than one possible answer.
Writing expressions in Radical form, and in exponential form
Choose convert to radical form from the topic selector and click to see the result in our algebra calculator ! Web write each expression in radical form. Sal rewrites (r^ (2/3)s^3)^2*√ (20r^4s^5), once as an exponential expression and once as a radical expression. There are no fractions in the radicand. Solution use the fact that n√an = a when n.
What is the expression in radical form?
We can also write the general rational exponent in terms of radicals as follows. Then, √a√b = √ab, and, provide b ≠ 0, √a √b = √a b in this section, we will simplify a number of more extensive expressions containing square roots, particularly those that are fundamental to your work in future mathematics courses. Sal rewrites (r^ (2/3)s^3)^2*√ (20r^4s^5),.
Activity 1 Exponential to Radical form and ViceversaRecall how to
1) 7 1 22) 4 4 3 3) 2 5 34) 7 4 3 5) 6 3 26) 2 1 6 write each expression in exponential form. Web simplifying radical expressions an algebraic expression that contains radicals is called a radical expression14. Solution use the fact that n√an = a when n is odd. The exponent of each factor of.
Writing Expression in Simplest Radical Form Geometry How to Help
Web a radical expression is said to be in standard form if the following conditions hold: Then, √a√b = √ab, and, provide b ≠ 0, √a √b = √a b in this section, we will simplify a number of more extensive expressions containing square roots, particularly those that are fundamental to your work in future mathematics courses. Enter the expression.
Write Each Expression In Radical Form printable pdf download
Solution use the fact that n√an = a when n is odd. = 3 ⋅ x = 3x. 3√27x3 = 3√33 ⋅ x3 applytheproductruleforradicals. 👉 learn how to convert a rational power to a radical. Web write each expression in radical form.
Write Each Expression In Radical Form, Or Write Ea...
When the exponent of an expression is a fraction, we can evaluate/simplify the expression by converting the rational power. Web a radical expression is said to be in standard form if the following conditions hold: The radical index is as small as possible. We use the product and quotient rules to simplify them. 7) (10)38) 6 2 9) ( 4.
Web Write Each Expression In Radical Form.
There are no fractions in the radicand. Then, √a√b = √ab, and, provide b ≠ 0, √a √b = √a b in this section, we will simplify a number of more extensive expressions containing square roots, particularly those that are fundamental to your work in future mathematics courses. Created by sal khan and monterey institute for technology and education. The radical index is as small as possible.
\[{A^{\Frac{M}{N}}} = {\Left( {{A^{\Frac{1}{N}}}} \Right)^M} = {\Left( {\Sqrt[N]{A}} \Right)^M}\Hspace{0.25In}\Hspace{0.25In}{\Mbox{Or}}\Hspace{0.25In}\Hspace{0.25In.
= 3 ⋅ x = 3x. We can also write the general rational exponent in terms of radicals as follows. 7) (10)38) 6 2 9) ( 4 2)510) (45)5 11) 3 2 12) 6 10 write each expression in radical form. When the exponent of an expression is a fraction, we can evaluate/simplify the expression by converting the rational power.
Web A Worked Example Of Simplifying An Expression That Is A Sum Of Several Radicals.
Let’s begin by building some fundamental skills. The exponent of each factor of the radicand is a natural number less than the radical index. Web property 9.4.1 let a and b be any two real nonnegative numbers. Solution use the fact that n√an = a when n is odd.
We Use The Product And Quotient Rules To Simplify Them.
In this example, we simplify √(2x²)+4√8+3√(2x²)+√8. Sal rewrites (r^ (2/3)s^3)^2*√ (20r^4s^5), once as an exponential expression and once as a radical expression. Web this is because there will never be more than one possible answer for a radical with an odd index. 3√27x3 = 3√33 ⋅ x3 applytheproductruleforradicals.
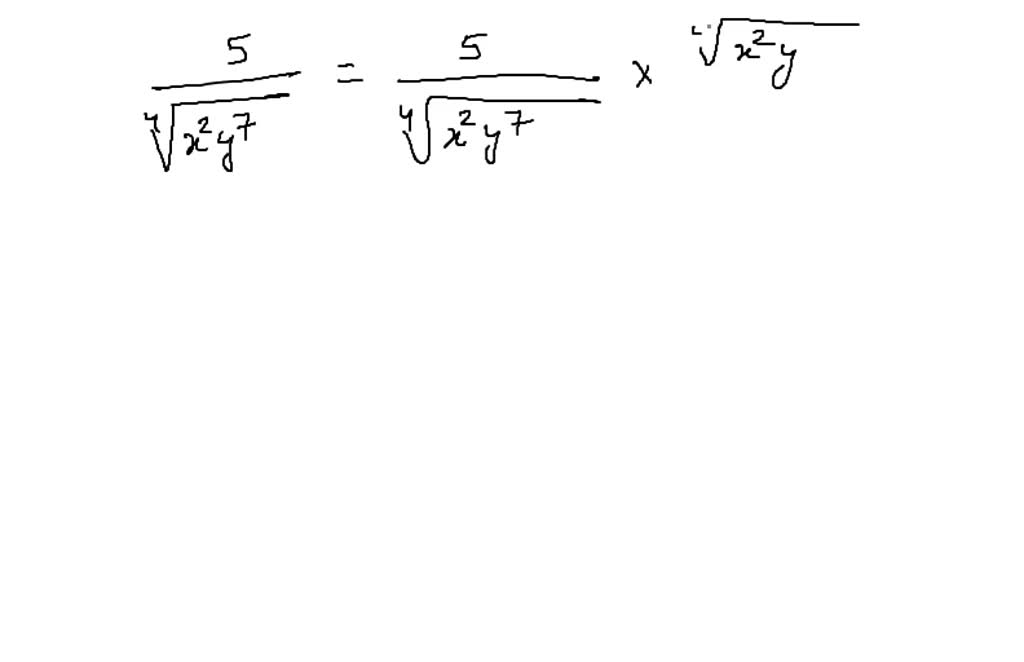
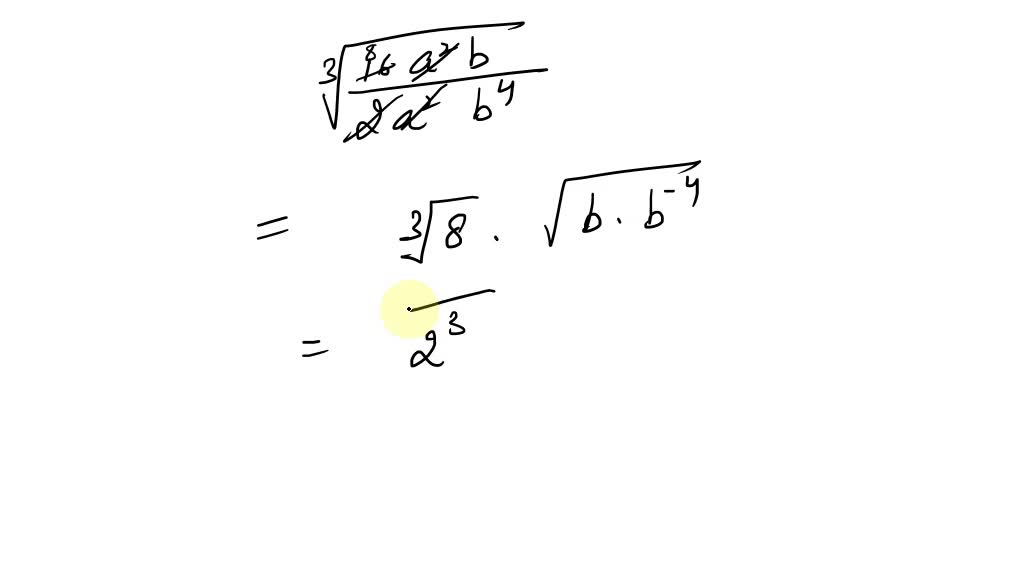
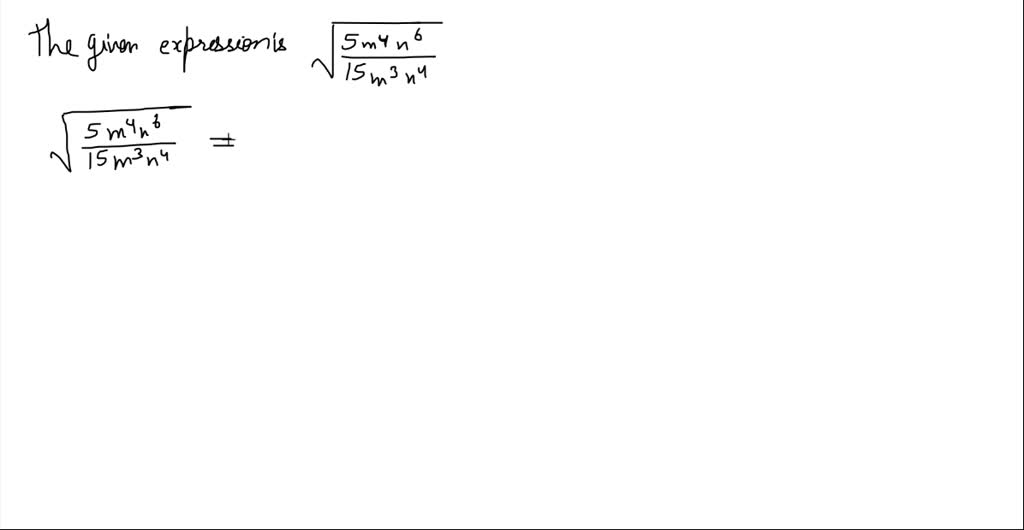
![[ANSWERED] Write each expression in radical form. Use "sqrt(x)" fo](https://media.kunduz.com/media/sug-question/raw/54751393-1658059626.431323.jpeg?h=512)