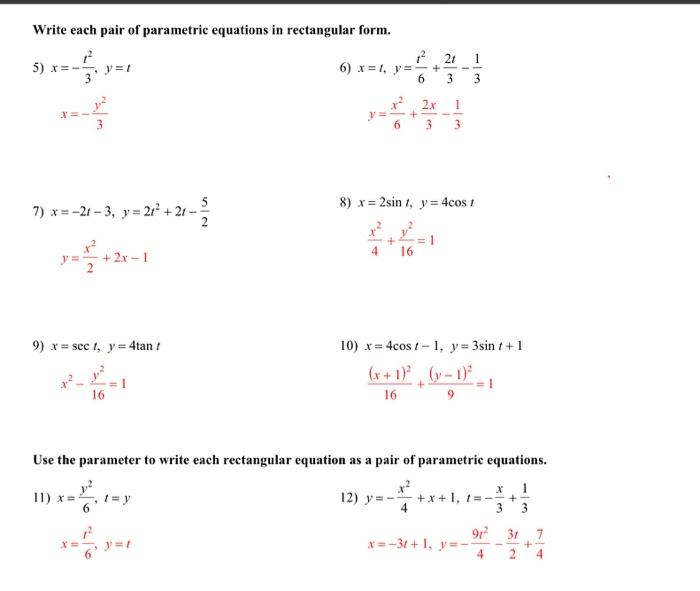
Write Each Pair Of Parametric Equations In Rectangular Form
Write Each Pair Of Parametric Equations In Rectangular Form - A) 1 25 25 y? 2 х + 15) x = 2cos t, y = 2sin t y? X = t2 x = t. Solution first, it is always possible to parameterize a curve by defining x ( t ) = t ,. Web parametric equations are a set of equations that express a set of quantities as explicit functions of a number of independent variables, known as parameters. for. B) 1 16 16 y? Then graph the equation and state any restrictions on the domain. Y = sec(t/2) now use the trig identity 1 + tan 2 θ = sec 2. Y 11) x x 12) y t x , y t t t Web write each pair of parametric equations in rectangular form.
Web find two different pairs of parametric equations to represent the graph of y = 2 x 2 − 3. 2 х + 15) x = 2cos t, y = 2sin t y? Write each pair of parametric equations in rectangular form. X = t2 x = t. Convert to rectangular x=t^2 , y=t^9. Write each pair of parametric equations in rectangular form. Web parametric equations are a set of equations that express a set of quantities as explicit functions of a number of independent variables, known as parameters. for. Web to convert the parametric equations to rectangular form requires doing the following. Web write each pair of parametric equations in rectangular form. You'll get a detailed solution from a subject matter expert that helps you learn core concepts.
A) 1 25 25 y? X = 1 log (t + 2) y = 2 t − 4 \begin{aligned} &x=\frac{1}{\log (t+2)}\\ &y=2 t. Write each pair of parametric equations in rectangular form. Web writing parametric equations in rectangular form. 2 х + 15) x = 2cos t, y = 2sin t y? Then state the restriction on the domain. A) 1 25 25 y? Follow along using the transcript. Write each pair of parametric equations in rectangular form. Y = sec(t/2) now use the trig identity 1 + tan 2 θ = sec 2.
Write the Parametric Equations of a Circle in Rectangular Form
Web up to 6% cash back parametric equations a rectangular equation, or an equation in rectangular form is an equation composed of variables like x and y which can be graphed on. A) 1 25 25 y? A) 1 25 25 y? X = t2 x = t. Web write each pair of parametric equations in rectangular form.
Converting Parametric Equation to Rectangular Form YouTube
Then graph the equation and state any restrictions on the domain. Y = sec(t/2) now use the trig identity 1 + tan 2 θ = sec 2. Web to convert the parametric equations to rectangular form requires doing the following. X = 2 t ± 5, y = t2 + 4 62/87,21 solve for t in the. Web writing parametric.
Rectangular Form Of Parametric Equations akrisztina27
A) 1 25 25 y? Web up to 6% cash back parametric equations a rectangular equation, or an equation in rectangular form is an equation composed of variables like x and y which can be graphed on. A) 1 25 25 y? Web writing parametric equations in rectangular form. Web write each pair of parametric equations in rectangular form.
Write the Parametric Equations x = ln(t), y = 3ln(t) in Rectangular
Convert to rectangular x=t^2 , y=t^9. X = t2 x = t 2 , y = t9 y = t 9. Web this problem has been solved! Then state the restriction on the domain. Then graph the equation and state any restrictions on the domain.
Rectangular Form Of Parametric Equations akrisztina27
Web write each pair of parametric equations in rectangular form. Solution first, it is always possible to parameterize a curve by defining x ( t ) = t ,. X = 1 log (t + 2) y = 2 t − 4 \begin{aligned} &x=\frac{1}{\log (t+2)}\\ &y=2 t. Write each pair of parametric equations in rectangular form. Web write each.
Rectangular Form Of Parametric Equations akrisztina27
Then state the restriction on the domain. Write each pair of parametric equations in. 23) x = 3sin2t+ 2ty = 4cos2t −1 24) x = − 5r2 ⋅y = t 25x +−4r− 3,3 = − 34e2 − 4t −3 26) x =. Web find two different pairs of parametric equations to represent the graph of y = 2 x 2.
How to convert parametric equations to rectangular form example 3 YouTube
Web to convert the parametric equations to rectangular form requires doing the following. B) 1 16 16 y? Web write each pair of parametric equations in rectangular form. A) 1 25 25 y? Y = 2 x 2 − 3.
SOLVEDFind a rectangular equation equivalent to the given pair of
Web write each pair of parametric equations in rectangular form. X = 1 log (t + 2) y = 2 t − 4 \begin{aligned} &x=\frac{1}{\log (t+2)}\\ &y=2 t. Web write each pair of parametric equations in rectangular form. Web up to 6% cash back parametric equations a rectangular equation, or an equation in rectangular form is an equation composed.
Rectangular Form Of Parametric Equations akrisztina27
Web write each pair of parametric equations in rectangular form. Web find two different pairs of parametric equations to represent the graph of y = 2 x 2 − 3. Y = sec(t/2) now use the trig identity 1 + tan 2 θ = sec 2. Then state the restriction on the domain. A) 1 25 25 y?
Rectangular Form Of Parametric Equations akrisztina27
Web writing parametric equations in rectangular form. Then state the restriction on the domain. Then graph the equation and state any restrictions on the domain. Convert to rectangular x=t^2 , y=t^9. 2 х + 15) x = 2cos t, y = 2sin t y?
Web Write Each Pair Of Parametric Equations In Rectangular Form.
Write each pair of parametric equations in rectangular form. Then state the restriction on the domain. X = 2 t ± 5, y = t2 + 4 62/87,21 solve for t in the. Write each pair of parametric equations in rectangular form.
X = T2 X = T 2 , Y = T9 Y = T 9.
Web write each pair of parametric equations in rectangular form. Web use the parameter to write each rectangular equation as a pair of parametric equations. Y = 2 x 2 − 3. You'll get a detailed solution from a subject matter expert that helps you learn core concepts.
X = T2 X = T.
Web parametric equations are a set of equations that express a set of quantities as explicit functions of a number of independent variables, known as parameters. for. Y 11) x x 12) y t x , y t t t 2 х + 15) x = 2cos t, y = 2sin t y? Web write each pair of parametric equations in rectangular form.
| Quizlet Related Questions With Answers Involve Trigonometric Equations Quadratic In Form.
Web find two different pairs of parametric equations to represent the graph of y = 2 x 2 − 3. A) 1 25 25 y? Web to convert the parametric equations to rectangular form requires doing the following. Web writing parametric equations in rectangular form.